The 2020 nobel prize was awarded to Sir Roger Penrose (one-half) for ‘the discovery that black hole formation is a robust prediction of the general theory of relativity’, and to Reinhard Genzel and Andrea Ghez (one-quarter each) for ‘the discovery of a supermassive compact object at the centre of our galaxy’. The prize recognised in equal parts the theoretical and experimental works that helped confirm the existence of black holes. Perhaps the first thought in most physicists’ minds when this year’s prize was announced was that Stephen Hawking might have won it had it been announced a few years sooner.
The term ‘black hole’ has been a commonly used one since it was first coined by John Wheeler in 1967. At that point, it had been only two years since the study of black holes had been kickstarted. A lot had happened by 1999 though, when, in his autobiography, Wheeler wrote that black holes teach us—
...that space can be crumpled like a piece of paper into an infinitesimal dot, that time can be extinguished like a blown-out flame, and that the laws of physics that we regard as ‘sacred,’ as immutable, are anything but...
John A. Wheeler, ‘Geons, Black Holes & Quantum Foam: A Life in Physics’, 1999.
A lot of these lessons came from the work of Sirs Roger Penrose and Stephen Hawking. Penrose in particular was the one who had kickstarted the rage about black holes in 1965 and developments have been made ever since right up to last year—half-a-century later—when we photographed a black hole for the very first time in history.
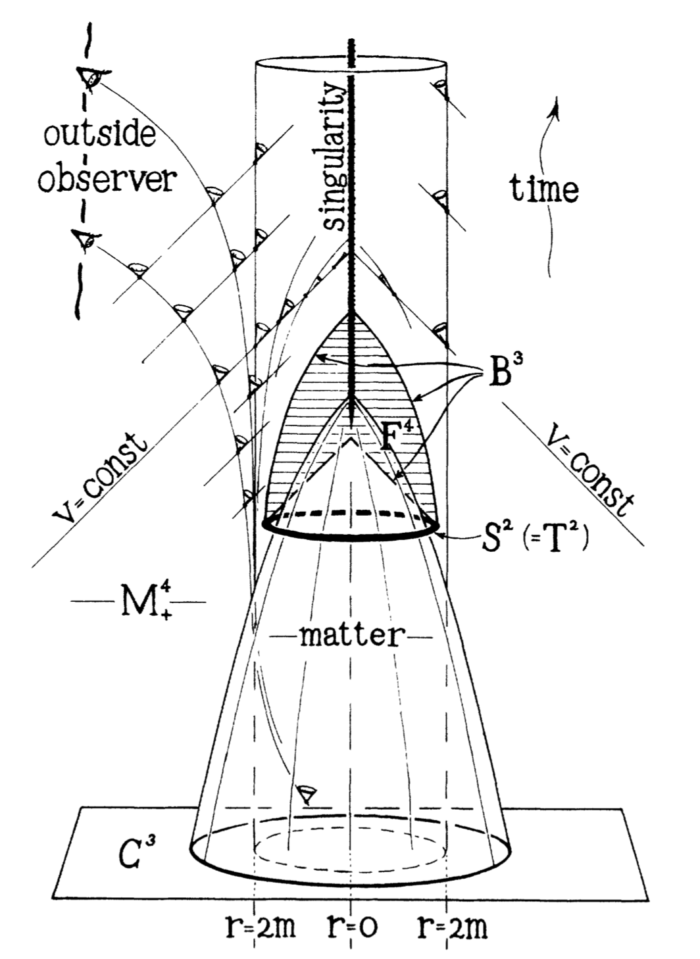
Black holes really do exist
In short, what Penrose’s work showed us was that, based on the equations of General Relativity, the occurrence of black holes was certainly a proper, consistent and highly predictable outcome. Those who have taken a class in astrophysics, cosmology or similar advanced physics courses will point out that it was in fact Karl Schwarzschild who gave several solutions to general relativity, one of which was what we now call a ‘black hole’. While this is true, the problem was that Schwarzschild’s solutions were idealistic. They would apply under ideal conditions which are convincing enough for theoreticl physicisits as they rarely compromise unreasonably on accuracy, but that were not practically convincing.
In the early-to-mid-1960s, Sir Roger Penrose began working on black holes—this was around the time he began his collaboration with Stephen Hawking—and eventually, in 1965, published his seminal paper, ‘Gravitational collapse and space–time singularities’. The paper put to rest a simple but effective discussion: while the consensus was that Schwarzschild’s solution worked in an ideal universe, Sir Roger’s work showed that this was not the case. He was ablse to prove half-a-century after Einstein published general relativity that star sufficiently massive would definitely collapse into a black hole and this was in the most practical sense, not ideally.
Worth trying to detect one
The evidence Sir Roger Penrose gave showed us that far from being needles in haystacks black holes were probably a more consistent occurrence than we had imagined earlier. They would not be found everywhere you pointed your telescope—and you could not actually ‘see’ them straightaway either with an optical telescope in your backyard—but there was now enough reason to warrant an attempt to track one or two of them down.
In 1996 a paper was published in Nature about the movement of stars close to the centre of our Milky Way galaxy. And by close we mean between 10,000 and 100,000 times the Earth–Sun distance. The paper traced the movement of these stars in infrared (as they were invisible in the optical region), circling around a common centre, and showed that their movement was consistent with the presence of an massive object at the centre of the galaxy. They suspected the mass of this object to be at least \(2.4 \times 10^6 M_{\odot}\) or nearly two-and-a-half million times the mass of our Sun (\(M_{\odot}\)). The lead author of that paper was Reinhard Genzel.
For sixteen years following this, Genzel led the team that studied the galactic centre. In 2009 they were able to present a refined estimate—now accepted to be accurate—of \(4.31 \times 10^6 M_{\odot}\), or about four million times the mass of the Sun. This could have been a group of stars, or even sets of binary stars, which is why it took a 16-year study to show beyond reasonable doubt that the only object that fit the model Genzel and his team had observational evidence for was simply a single point-mass. A black hole.
At last we had plenty of observational evidence for this now, which led Genzel to famously proclaim back then that the 2009 study gave ‘the best empirical evidence that supermassive black holes do really exist.’ He continued, ‘The stellar orbits in the Galactic Center show that the central mass concentration of four million solar masses must be a black hole, beyond any reasonable doubt.’ We now call this black hole Sagittarius A* and it is the supermassive black hole we now know lies at the centre of our own galaxy.
Genzel’s work studying the galactic centre goes on to this day, and while it would be relishing to touch upon it all, that would exceed the scope of this report. We will therefore move on to our third and final Nobel laureate of the year.
More IR star tracking
Recall how Genzel and co and worked on tracking stellar orbits about the centre of the Milky Way? Two years later Andrea Ghez heading the UCLA Galactic Centre Group oversaw more or less the same measurements but with a huge increase in accuracy thanks to quicker bursts of recording that helped them clear out any fuzziness arising due to stellar atmospheres being dragged across their image sensors.
However, Ghez’s group did one thing differently: they measured select stars near the galactic centre without the assumption that there existed a common orbital point in the centre. What they found was quite surprising: measurement of stellar velocities showed that the stars must be adhering to a Keplerian orbit, which meant—like the planets in our solar system taking Keplarian orbits around the Sun—the stars at the centre of the Milky Way must be orbiting some object located at the centre.
Ghez’s group realised that one of these stars, which they called S0-2 (S because of its location in Sagittarius and 2 because it was the second closest star to the galactic centre when discovered), had an orbital period of about 15 Earth years, which meant they could follow it continuously. Aided by some advanced image processing systems (adaptive optics) the group measured the same galactic central stars for over a decade.
In 2018, the star known as S0-2 reached its pericentre, or the point of closest orbit, at 2.55% the speed of light. This was a key moment for the group: at such close distances to a massive star, if there was one, general relativity predicts that light from the star will undergo combined gravitational redshift and Doppler redshift. Both Ghez’s group and Genzel’s group measured that this characteristic redshift did in fact occur. This not only proved general relativity, but also cleared any doubts that the object at the centre of our milky way was a supermassive blackhole.
As a side note, Andrea Ghez’s winning the Nobel prize makes it two people who have worked on the accurate depiction of black holes in the film Interstellar and then gone on to win Nobel prizes. (The other was Kip Thorne in 2017.)
Looking forward
It was a unique year for the physics Nobel prize because of how both theoretical and experimental physics shared the stage. While Sir Roger Penrose’s work got the ball rolling for modern-day studies of black holes, Reinhard Genzel’s and Andrea Ghez’s work—each closely mirroring the other—proved the existence of a black hole about as close to home as one might expect.
The awards may have rolled in but the journey is far from over. Both the MPE and the UCLA Galactic Center Group continue to collect data on these stars to this day in hopes that soon they will be able to provide us an even better understanding of our galaxy, of relativity, of black holes, and in the end of our universe.