There is an old joke in physics: topologists cannot tell their pretzels from their coffee cups. This interesting, almost quirky, branch of mathematics—which sees considerably use in physics—won this year's Nobel prize for three British physicists, Michael Kosterlitz, Duncan Haldane, David J. Thouless. The prize was awarded for the ‘theoretical discoveries of topological phase transitions and topological phases of matter’ by the Royal Swedish Academy of Sciences.
While Nobel committee member and physicist, Thors Hans Hansson, did a great job of explaining it with a bun, a bagel and a Swedish pretzel, much to the entertainment, and education, of the media, we would like to re-visit this with an explainer that goes slightly deeper into the topic, and that may or may not involve more talk about food.
An issue of condensed matter
There were two recurring gags throughout my graduate years in physics: first, was that condensed matter physics wins every other Nobel prize (as it did this year), and second, that a conference on time-travel will be held two weeks ago. Most physicists know this. Most physicists who studied condensed matter physics will also know that a considerable chunk of it deals with what are called phase transitions .
There is no shortage of bizarre effects in this area of physics. There is, especially, physics around absolute zero (or about –273ºC, which is the lowest possible temperature) where, as we tend to this temperature, a number of interesting phenomena occur, most notably superconductivity and superfluidity. Superconductivity is is extremely high current flow with practically no resistance, and superfluidity is analogously the flow of any fluid with practically zero viscosity. However, these effects, given the initial understanding we had of them around the late 20th century, were not believed to exist in thin, film-like, almost (but not quite) two-dimensional materials.
Drs Kosterlitz and Thouless had, by the 1970s, used topology to explain how such effects can occur in such thin, near-2D films of select materials as well. This was, first of all, heralded as a great binder of pure mathematics and modern physics because they had used topology to describe this. And topology was, as we shall soon see, more of an abstract, mathematical concept without any immediate applications in the real world besides modelling. With the work of Drs Kosterlitz, Thouless and Haldane, not only has topology been exploited in physics, but it is expected to, through this, see use in several futuristic avenues like quantum computing.
Phase transitions
Phase transitions are an important part of condensed matter physics. Matter in general exists in four main forms, or states , or phases : solid, liquid, gas, and plasma. The transition we refer to is the change a substance undergoes as it goes from being a solid to becoming a liqiud, or from a liquid to a gas and so on.
Each phase is described by an equation known as the equation of state , which is continuous in a given phase—which simply means it exists everywhere, for any value of the thermodynamic variables of pressure, temperature etc. associated with that phase. However, these equations are discontinuous during a phase transition, such as when a solid becomes a liquid or a liquid becomes a gas.
This discontinuity can be of two types, mathematically. For a parameter called the Gibbs free energy , or simply the amount of energy a body has ‘free’ for use to perform some work, the variation of free energy (\(G\)) with any thermodynamic variable (say pressure, \(P\), or temperature, \(T\)), is given by \(\textrm{d} G/\textrm{d} V\) or \(\textrm{d} G/\textrm{d} T\) and so on. If this is discontinuous between the two phases, i.e. during the phase transition, then we call it a first-order phase transition . If this discontinuity is in the second derivative of \(G\), then the transition is called a second-order phase transition .
XY model and symmetries
As we slowly delve into higher, purer physics, it becomes that much more difficult to put it into simpler words without dumbing things down. We will, therefore, have to settle for a delicate balance: atoms in crystals are arranged periodically in space to form what is called a lattice . They can be thought of as repeated arrangements of a single pattern of a bunch of atomic or molecular placements that can be placed adjacent, in all directions, over and over again, to form the entire crystal—ideally speaking, that is, because in reality some defects creep into the picture, but we will not concern ourselves with that for now.
One popular lattice model is called the classical rotor model, or the XY model. It is two-dimensional and circularly symmetric. And one of its properties is that it does not exhibit second-order phase transitions. There is, however, a special type of phase transition that occurs between the phases of a material possessing such an XY lattice, and is called the Kosterlitz-Thouless transition after Drs Kosterlitz and Thouless.
A primer on topology
Topology is a branch of mathematics that has to do with the surface of materials. Being the only differentiator, it means a lot of visually and chemically dissimilar materials or objects are topologically the same thing. For example, a coffee mug and a doughnut (yes, in reference to the old joke) both have one hole—the coffee mug in its handle and the doughnut right in its centre. Topologically, this means they are the same.
More precisely, the mug and the doughnut have spatial properties that are preserved under continuous deformation. You can bend the mug (albeit not without some planning and effort) to make it visually similar to a doughnut—and vice versa. Anything works so long as you are not pulling things apart or sticking them together. This is called homeomorphism . There are others like it that are increasingly more complex and we will not delve into them for now.
The takeaway here is that a classification of a “space” which is homeomorphic has topological properties that remain unchanged throughout the process of homeomorphism—coffee mugs becoming doughnuts retain their property of having only one hole—and such properties are called topological invariants .
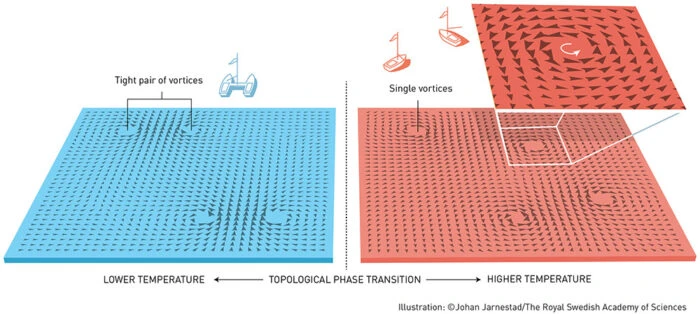
KT transition
Back the late 1940s, Lars Onsager and others had theorised that a topological defect or soliton (note that the term defect here simply means there is a mathematical solution different from the ideal vacuum case) such as a vortex could explain phase transitions in superfluids. Called quantum vortices (see picture above), their existence did not make a (nearly) two-dimensional substance unstable in any way. At this magnification, the vortices occur as a result of electron spins and can be visualised in much the same manner as any regular vortex in water.
The general idea in 70s was that any 2D film (once again, this is almost 2D since we neglect the height contributed by sheets of atoms themselves) would not survive long thanks to thermodynamics, which in turn meant that ordered phase transitions were out of the question. Drs Kosterlitz and Thouless found, however, that they could attribute conductance to the topological idea of vortices themselves and not simply as an analogue of current flow in 3D wires. This was the first example of bringing topology into condensed matter physics and also of phase transitions occurring with no clearly ordered phase. At low temperatures, these vortices can be shown to be tightly paired. And as temperatures increase, they drift apart allowing for phase transitions from one state of matter to another, mathematically parallel to the ones we are already aware of.
Since little can be explained without invoking the Hamiltonian of an XY model, you will have to take our word for it: the Kosterlitz-Thouless phase transition is an infinite order transition which, although initially applied to ideal 2D surfaces, found its way over time to 3D models as well, seeing promising use in everyday technology. The beauty of this is that, somewhat like Bose-Einstein condensates, anything that helps understand phase transitions sees great use across physics: from cosmology, where the evolution of the universe is studied in terms of phase transitions like in condensed matter physics, all the way to high energy particle physics, this has implications almost everywhere.
The quantum Hall effect
This year's physics Nobel was also in recognition of the explanation of the quantum Hall effect. Dr Thouless worked with Dr Haldane on this field in the 80s (which is why Dr Thouless won half the Nobel, while Drs Kosterlitz and Haldane shared the other half).
Suppose you had a slim, cuboidal piece of some conductor material and you placed it in a magnetic field breadthwise and passed current lengthwise along it (so that the current and magnetic field are perpendicular to each other), you would soon observe that a voltage develops across the conductor, perpendicular to both the direction of the magnetic field and the supplied current. Think of each (current, magnetic field, developed voltage) as being along one of three x, y, z axes. This voltage is called the Hall voltage and the effect itself is named after its discoverer, Edwin Hall. This was around 1879.
Nearly one hundred years later, the german physicist, Klaus von Klitzing, while studying a thin, (once again almost but not quite) two-dimensional conductor placed in an extremely low temperature and an extremely high magnetic field, found that the Hall voltage was not continuous, i.e. it did not take any which value, and instead only took values in certain discrete steps—or, as physicists would say, it was quantised . He won the Nobel prize just five years later, in 1985, for this discovery.
Specifically, von Klitzing found the Hall resistance (due to the supplied current and the consequent Hall voltage) to be quantised in steps of $h/ne^2$, with $h$ being Planck's constant, $e$ the charge of an electron, and $n$ the filling factor which was experimentally found to take only selective values: $\frac{1}{3}$, $\frac{2}{5}$, $\frac{3}{7}$ ... $1$, $2$, ... $\frac{12}{5}$ and so on. The mystery, for almost the next decade, was why $n$ was selective in this manner.
A topological toolkit—and its implications
The answers to all this lay in Dr Thouless's and Dr Haldane's work in the ’80s. They were able to show, using topology, that the theory could agree with von Klitzing and others' observations. Dr Thouless was mathematically able to prove that the atoms behave like what is called a ‘topological fluid’, moving in discrete steps that stemmed from topology—the discrete steps were, in a manner of speaking, the topological invariant.
Dr Haldane was able to extend this to higher dimensions based on his work with magnetic chains of atoms where he had shown that even atoms obeyed topology and odd ones did not, and that they broke inversion symmetry. He was able to show that, based topologically on his explanation of a single chain of magnetic atoms, such effects as the ones Dr Thouless had used to explain the quantum hall effect in two-dimensional sheets could be observed in higher dimensions as well, and in semiconductors even in the absence of a magnetic field.
The use of topology in physics has since seen a huge increase. It is commonly used today across fields: condensed matter physicists, high-energy physicists and cosmologists all use it. On the practical side of things, this has led to interesting materials such as topological insulators that can conduct on their surface and insulate within, and topological superconductors and other techniques which are proving to be successes in quantum computing but are yet to enter the mainstream.
This year's prize was particularly for the theoretical work that explained several unexplained experimental observations, some of which (like von Klitzing's) which had won a Nobel already. The effects of their work are being felt and will continue to be felt for a long time, as the Nobel committee put it: ‘Current research is revealing the secrets of matter in the exotic worlds [were] discovered by this year’s Nobel Laureates.’
